Learning how to divide fractions might seem intimidating at first, but trust me, it’s a piece of cake once you get the hang of it. Fractions are everywhere—in recipes, measurements, and even in your daily life. Whether you're a student trying to ace math or an adult brushing up on forgotten skills, understanding how to divide fractions is essential. So, buckle up, because we’re diving deep into the world of numerators and denominators!

Now, I know what you're thinking—math can be scary, right? But don’t worry, I’ve got your back. This guide is designed to break down the process step by step, so you can master dividing fractions like a pro. By the time you’re done reading, you’ll be solving fraction problems faster than you can say "pi"!

So, why is learning how to divide fractions so important? Well, it’s not just about passing a test or acing a quiz. Dividing fractions helps you in real-life situations, like splitting a pizza among friends, calculating discounts, or even figuring out how much paint you need for a room. Sound useful? Let’s get started!

Read also:
Camilla Araujo Pron

Understanding Fractions: The Basics

Before we dive into how to divide fractions, let’s take a quick refresher on what fractions actually are. A fraction represents a part of a whole. It consists of two parts: the numerator (the top number) and the denominator (the bottom number). Think of it as a way to divide things into smaller, equal parts. For example, if you have a pizza cut into 8 slices and you eat 3 slices, you’ve eaten 3/8 of the pizza. Simple, right?

Types of Fractions

Not all fractions are created equal. There are three main types of fractions:

Proper Fractions: The numerator is smaller than the denominator (e.g., 1/2).
Improper Fractions: The numerator is larger than or equal to the denominator (e.g., 5/4).
Mixed Numbers: A combination of a whole number and a fraction (e.g., 2 1/3).

Knowing these types will help you understand how to divide fractions more easily. Now that we’ve got the basics covered, let’s move on to the fun part!

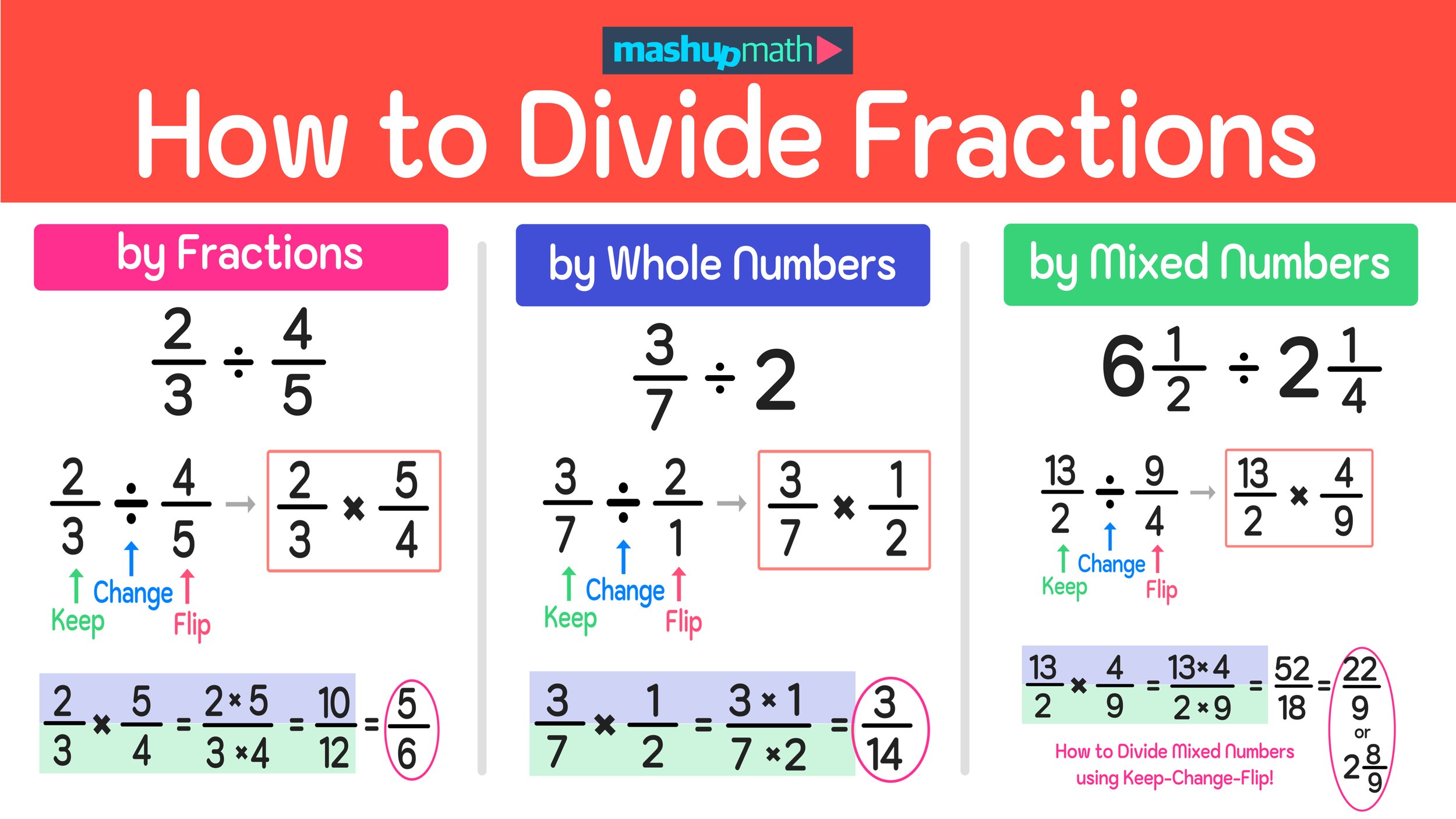
How to Divide Fractions: Step by Step

Dividing fractions might sound tricky, but it’s actually quite simple when you follow the right steps. Here’s how you do it:

Flip the second fraction (the divisor) to create its reciprocal.
Multiply the first fraction by the reciprocal of the second fraction.
Simplify the resulting fraction if possible.

Let’s break it down further with an example. Say you want to divide 1/2 by 1/4:

The reciprocal of 1/4 is 4/1.
Multiply 1/2 by 4/1, which gives you 4/2.
Simplify 4/2 to get 2.

See? It’s not that hard when you break it down step by step!

Read also:
Funkytown Gore

Common Mistakes to Avoid When Dividing Fractions

Even the best of us make mistakes when dividing fractions. Here are a few common ones to watch out for:

Forgetting to flip the second fraction.
Not simplifying the final answer.
Multiplying instead of dividing (or vice versa).

Pay attention to these pitfalls, and you’ll save yourself a lot of headaches. Trust me, I’ve been there!

Why Simplifying Matters

Simplifying fractions might seem like an extra step, but it’s crucial for getting the correct answer. For example, if you end up with 6/8, simplifying it to 3/4 gives you a cleaner, more accurate result. It also makes your work easier to understand for others. Who doesn’t love a tidy fraction?

Dividing Mixed Numbers: A Special Case

What happens when you’re dividing mixed numbers? Don’t panic—it’s easier than it sounds. Here’s how you handle it:

Convert the mixed numbers to improper fractions.
Follow the same steps for dividing fractions.
Simplify the result if needed.

For instance, if you’re dividing 2 1/3 by 1 1/2:

Convert 2 1/3 to 7/3 and 1 1/2 to 3/2.
Flip the second fraction to get 2/3.
Multiply 7/3 by 2/3, which gives you 14/9.
Convert 14/9 back to a mixed number if preferred (1 5/9).

See? Not so bad, right?

Practical Applications of Dividing Fractions

Dividing fractions isn’t just a math exercise—it has real-world applications. Here are a few examples:

Cooking and Baking: Adjusting recipes for larger or smaller groups.
DIY Projects: Calculating measurements for wood, fabric, or paint.
Finance: Splitting bills or dividing profits among partners.

Understanding how to divide fractions can make these tasks much easier and more accurate. Who knew math could be so practical?

Dividing Fractions in Everyday Life

Let’s say you’re baking a cake, and the recipe calls for 3/4 cup of sugar, but you only want to make half the recipe. How much sugar do you need? Divide 3/4 by 2, and you get 3/8 cup. Easy peasy!

Advanced Techniques for Dividing Fractions

Once you’ve mastered the basics, you can move on to more advanced techniques. For example, try dividing fractions with variables or complex numbers. It’s like leveling up in a video game!

Here’s an example: Divide (x/3) by (2/y). First, flip the second fraction to get (y/2). Then multiply (x/3) by (y/2), which gives you (xy/6). Voilà! You’ve just entered the world of algebraic fractions.

Tips for Solving Complex Fraction Problems

Solving advanced fraction problems requires a bit more brainpower, but these tips will help:

Break the problem into smaller steps.
Use cross-multiplication when necessary.
Double-check your work for accuracy.

Remember, practice makes perfect. The more you work with fractions, the better you’ll get at solving even the trickiest problems.

How to Teach Dividing Fractions to Kids

Teaching kids how to divide fractions can be a rewarding experience. Here are some tips to make it fun and engaging:

Use visual aids like fraction circles or bars.
Relate fractions to real-life situations, like sharing snacks.
Encourage hands-on activities, such as cutting paper into equal parts.

Kids learn best when they’re having fun, so don’t be afraid to get creative. Who knows? You might even enjoy teaching them!

Games and Activities for Learning Fractions

Here are a few games and activities to make learning fractions more enjoyable:

Fraction Bingo: Create bingo cards with fractions instead of numbers.
Fraction War: Use a deck of cards to play a fraction version of the classic card game.
Cooking Challenge: Have kids follow a recipe that requires dividing fractions.

These activities not only teach fractions but also build confidence and problem-solving skills.

Conclusion: Mastering How to Divide Fractions

Dividing fractions doesn’t have to be scary. With the right approach and a bit of practice, you can become a fraction division expert in no time. Remember to:

Flip the second fraction to create its reciprocal.
Multiply the first fraction by the reciprocal.
Simplify the result if possible.

Whether you’re a student, a teacher, or just someone looking to brush up on math skills, learning how to divide fractions is a valuable skill. So, go ahead and give it a try. You’ve got this!

Now, here’s the fun part: leave a comment below and let me know how dividing fractions has helped you in your daily life. Or, share this article with a friend who could use a math refresher. Together, we can make math less intimidating and more approachable for everyone!